iBet uBet web content aggregator. Adding the entire web to your favor.
iBet uBet web content aggregator. Adding the entire web to your favor.



Link to original content: https://www.ncbi.nlm.nih.gov/pmc/articles/PMC4632618
Hummingbird tongues are elastic micropumps - PMC Skip to main content
PMC home page
Proceedings of the Royal Society B: Biological Sciences logoLink to Proceedings of the Royal Society B: Biological Sciences
. 2015 Aug 22;282(1813):20151014. doi: 10.1098/rspb.2015.1014

Hummingbird tongues are elastic micropumps

Alejandro Rico-Guevara 1,3,✉, Tai-Hsi Fan 2, Margaret A Rubega 1
PMCID: PMC4632618  PMID: 26290074

Abstract

Pumping is a vital natural process, imitated by humans for thousands of years. We demonstrate that a hitherto undocumented mechanism of fluid transport pumps nectar onto the hummingbird tongue. Using high-speed cameras, we filmed the tongue–fluid interaction in 18 hummingbird species, from seven of the nine main hummingbird clades. During the offloading of the nectar inside the bill, hummingbirds compress their tongues upon extrusion; the compressed tongue remains flattened until it contacts the nectar. After contact with the nectar surface, the tongue reshapes filling entirely with nectar; we did not observe the formation of menisci required for the operation of capillarity during this process. We show that the tongue works as an elastic micropump; fluid at the tip is driven into the tongue's grooves by forces resulting from re-expansion of a collapsed section. This work falsifies the long-standing idea that capillarity is an important force filling hummingbird tongue grooves during nectar feeding. The expansive filling mechanism we report in this paper recruits elastic recovery properties of the groove walls to load nectar into the tongue an order of magnitude faster than capillarity could. Such fast filling allows hummingbirds to extract nectar at higher rates than predicted by capillarity-based foraging models, in agreement with their fast licking rates.

Keywords: capillarity, feeding mechanism, fluid dynamics, hummingbird foraging

1. Introduction

Hummingbirds have remarkably high metabolic rates, amazing speed and superb aeronautic control. All these traits result from the ability of hummingbirds to feed on nectar efficiently enough to fuel an extreme lifestyle out of a sparse, but energetically dense, resource. Therefore, the way in which they feed on nectar determines the peaks and span of their performance, and thus their behaviour (and evolutionary trajectory), across a range of environmental axes. Accordingly, the details of their feeding ecology and coevolution with flowers have been the subject of intense study for over 40 years [1–6]. Half a century's worth of coevolutionary theory, as understood through hummingbirds as an example, depends on obtaining an empirical and mechanistic understanding of the nectar collection process.

The hypothesis that capillarity moves the nectar inside hummingbird tongues has been proposed as an inference arising from their anatomy (e.g. [7]) and fairly limited in vivo experimental data [8]. The idea that capillarity is important in nectar feeding is intuitively attractive and understandable, but lacks evidence in extensive field investigations. The tongue of a hummingbird features paired longitudinal grooves running from near the tip to mid-tongue (figure 1); these grooves in their relaxed state resemble open cylinders, which is the reason why meniscus-driven (capillarity) equations have been invoked. Capillarity equations have been used to infer optimal concentrations in the nectar produced by bird-pollinated plants [9–11], optimization in drinking behaviours of nectar-feeding animals [12] and fluid transport optimality in a variety of natural and artificial systems [13]. However, during five years of high-speed filming of free-living hummingbirds, we accrued the most comprehensive dataset of tongue–nectar interactions reported to date, and both our qualitative and quantitative analyses show that capillarity cannot account for either the tongue dynamics, or for the rate at which nectar fills the grooves in unmanipulated hummingbirds feeding in the wild.

Figure 1.

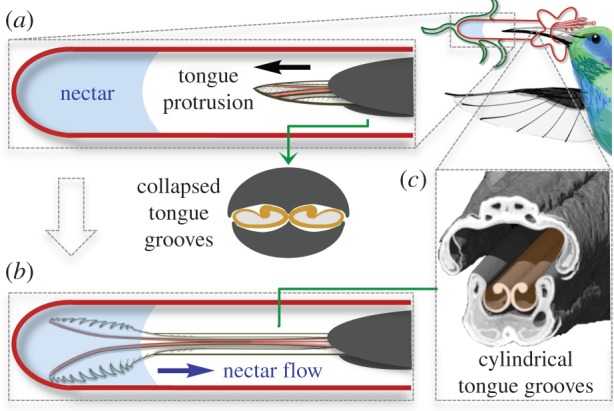
Figure 1.

Open in a new tab

The hummingbird tongue fills with nectar even when only the tip is immersed. (a) Hummingbirds can drink from flowers with corollas longer than their bills by extending their bifurcated, longitudinally grooved tongues to reach the nectar. During protrusion, the tongue is compressed as it passes through the bill tip, which results in a collapsed configuration of the grooves (cross-section). (b) Upon reaching the nectar, the tongue tips fringed with lamellae roll open and spread apart, but some of the grooved portions of the tongue will never contact the nectar pool. For the grooves to fill with nectar, they must return to their uncompressed, cylindrical configuration. (c) Coronal cutaway from a µCT scan showing the bill and tongue architecture. Hummingbird drawn by K. Hurme. (Online version in colour.)

2. Mutually exclusive possibilities

Hummingbirds extract nectar from flowers by licking—they repetitively protrude their tongue (figure 1a), dip the tip into the pool of nectar inside a flower (figure 1b), and then retract the tongue. During protrusion, they use their bill tips to squeeze the nectar off the tongue grooves inside the bill [14]. Consequently, as the tongue emerges, it is compressed along the grooves' entire length [15]. Just exterior to the compression point (the bill tip), the grooves exhibit a collapsed configuration (figure 1a). Once a given portion of the grooves passes the compression point, there are two possible, mutually exclusive, outcomes: (i) In the absence of the compression imposed by the bill tip over the tongue, the grooves could recover their shape, yielding two empty cylinders soon after the tongue emerges from the bill and before the tongue tips contact the nectar; or (ii) the grooves could remain collapsed while traversing the air, either by the force at the compression point being transferred along the length of the tongue walls or by the thin layer of liquid remaining inside the flattened grooves acting as an adhesive, or a combination of both.

The first scenario is compatible with the capillarity hypothesis [7–9]. If the tongue grooves reshape after compression, they will reach the nectar surface as two open cylinders (figure 1c) and can fill via capillarity. Since the grooves are open-sided, rather than complete cylinders, we expect that surface tension in the nectar will tend to ‘zip up’ the gap [8] as the fluid rises into the grooves. Capillarity (or ‘capillary suction’ sensu [8,9]) is a filling mechanism that operates in a tube or tube-like structure and which requires that such a structure contain an empty space that can be filled by a moving meniscus. This process can be observed quite easily, and the filling rate can be measured by tracking the moving meniscus. The expectation of a capillary process in the case of hummingbirds requires, therefore: (i) the existence, prior to filling, of an empty space in the tongue, (ii) an observable meniscus, and (iii) that the capacity of the whole structure should remain constant throughout the process. Models [8] propose that capillary suction fills the (i) empty space in the grooves in a hummingbird's tongue by slightly closing the tongue walls while the (ii) meniscus is passing through. The only difference in expectation between this and capillarity occurring in small glass tubes is that the walls of hummingbird tongue grooves are not static, but flexible, and the (iii) expectation is that the capacity of the structure should decrease slightly as it zips up (i.e. the groove closes around the fluid as the meniscus passes through). Thus, the capillarity hypothesis predicts that the section of the tongue composed of the grooves should decrease (or remain unchanged [9]) in thickness after filling, as compared to before contact with the nectar.

Conversely, if tongue grooves remain flattened before filling, then the condition of the tongue is incompatible with capillarity, since the rise of nectar into the grooves through capillarity requires that the grooves reshape into open cylinders before contact with the nectar. If the tongue grooves remain flattened until the tongue tip reaches the nectar surface, there is no empty cylinder and therefore no meniscus formation. In this scenario, capillarity is precluded by the geometry of the flattened tongue; if no menisci are formed, then capillarity equations (which track the velocity of the meniscus) are not applicable.

3. Results

(a). Capillarity is not biologically relevant to feeding in wild hummingbirds

Analysis of our videos demonstrates that capillarity is not operating during tongue-filling in free-living hummingbirds. Our observations and measurements from 96 foraging bouts, comprising hundreds of licks, of 32 birds of 18 species (electronic supplementary material, table S1) show that the tongue grooves remained collapsed until the tongue tips contacted the nectar surface (electronic supplementary material, video S1). After contacting the surface, the grooves expanded and filled completely with nectar (e.g. figure 2a). Formation of a meniscus within the tongue grooves was almost never observed (see theoretical versus experimental data section). This result is contrary to requirements for capillary filling (tongue grooves contacting the nectar surface as empty cylinders, and formation of a meniscus). Furthermore, measured tongue thicknesses did not conform to that predicted by capillarity filling the grooves; rather than decreasing (or remaining unchanged) as compared to before filling, tongue thickness increased (Student's paired t-test: p < 0.001, figure 2a, e.g. electronic supplementary material, videos S1 and S2). We calculated the percentage of groove expansion out of the final thickness of the groove. This percentage ranged from 48 to 60% among species (electronic supplementary material, table S1). All observed licks followed the same pattern: tongue thickness was stable during protrusion of the tongue, and rapidly increased after the tongue tips contacted the nectar (electronic supplementary material, video S2). After complete loading, the grooves filled with nectar were brought back inside the bill and squeezed for the next cycle, all in less than a tenth of a second.

Figure 2.

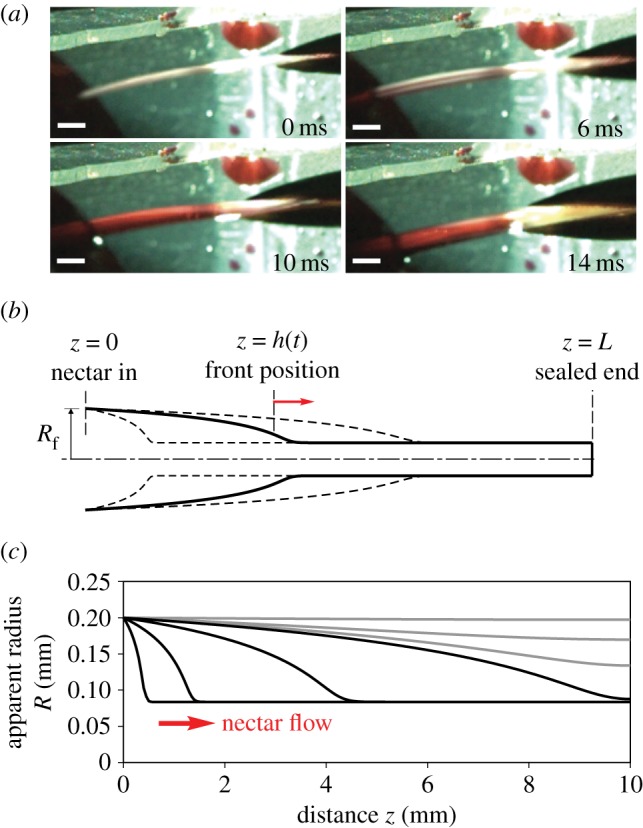
Figure 2.

Open in a new tab

Expansive filling mechanism. (a) Video frames showing a lateral view of an Amazilia hummingbird's tongue being protruded and contacting dyed red nectar. The full filling of the grooves is completed in 14 ms. Scale bars (white) = 0.5 mm. (b) A schematic showing the gradually expanded tube (groove) profiles and the position of the fluid front to be compared with experimental data. During the nectar filling process, the groove is wider near the liquid and thinner near the bill tip, the inverse of what would be expected if capillarity were filling the groove. (c) Axi-symmetric modelling results showing the transient profiles (apparent groove radius along its length) at 0.001, 0.01, 0.1 and 0.5 (black lines), and at 0.7, 1.0 and 2.0 (grey lines) times the characteristic time scale 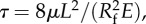Inline graphic with the parameters described in the text. Black lines describe progressive wave-like behaviour, and grey lines show the diffusive recovery. (Online version in colour.)

Summarizing and contrasting to the mutually exclusive hypotheses outlined in the introduction, we found that: (i) the tongue is, and remains, compressed prior to actual transport, and there is therefore no empty space within the tongue available to fill via capillarity; (ii) there is no meniscus during transport of fluid into the tongue; and (iii) tongue volume increases during transport, in contradiction of all the expectations for basic capillarity (e.g. no change in capacity) and the capillary suction model [8] (e.g. reduction in tongue volume). Thus, in free-living birds, feeding under realistic conditions, no expectations of either the traditional or the capillary suction model are met, thus we conclude that free-living birds are not using capillarity. These results on their own refute the idea that capillarity is an important component of the process that occurs while hummingbirds drink nectar.

(b). Novel fluid flow mechanism: a conceptual explanation

Our results, drawn from an unprecedentedly large and direct set of observations of the interaction of tongue and nectar, contradict the capillarity hypothesis [7–9] of drinking dynamics in hummingbirds. Some other process must account for the movement of fluid into the tongue grooves. Based on our observations of the tongue–fluid interactions in our videos, we offer here an alternative conceptual explanation, and a quantitative model (below), for the nectar uptake mechanism in hummingbirds. We incorporate the elastic recovering force on the grooves into a new nectar flow model, which correctly predicts the empirical data from feeding birds. The model, although with the limitations of any first approximation, provides a replacement for the capillarity equations that have been used to understand the energetics and ecology of hummingbird feeding [9–11].

We suggest that while squeezing nectar off the tongue during protrusion, the bird is collapsing the grooves and loading elastic energy into the groove walls that will be subsequently used to pump nectar into the grooves. The collapsed configuration is conserved during the trip of the tongue across the space between the bill tip to the nectar pool. Once the tongue tips contact the nectar surface, the supply of fluid allows the collapsed groove to gradually recover to a relaxed cylindrical shape until the nectar has filled it completely; hereafter, we refer to this previously undocumented mechanism as ‘expansive filling’. The liquid column has a progressive front within the tongue h(t) (figure 2b). We modelled a single uptake event by a simplified tube configuration with a sealed end at the groove base (figure 2b), and deduced that the fluid motion and uptake rate can be characterized by short- and long-time processes (figure 2c) based on the balance of inertial, elastic, viscous, and transmural pressure forces. The local effects of gravity are negligible owing to the small dimension of the system. The fluid is assumed to be Newtonian and incompressible in both short- and long-time processes.

(c). Elastohydrodynamic model of expansive filling

Considering a long-time quasi-steady and almost fully developed nectar flow in the elastic tube (groove) with a length of about 10–13 mm and inner diameter of 0.3–0.4 mm, the typical viscous incompressible fluid motion within a deformable tube is governed by a quasi-Poiseuille flow, which gives the local volumetric flow rate that is based on a linear relationship with the pressure gradient:

(c). 3.1

where tL indicates that this is a long-time process in which the inertia of the tube is neglected, the flow is approximately fully developed, and the elastic recovery of the tube is quasi-static, R is the apparent local radius of the tube, μ is dynamic viscosity, p is pressure, and the travelling distance of the liquid column is defined by 0 ≤ z ≤ L, where L is the tube length (much larger than the tube diameter). The flow rate and various apparent radii along the tube satisfy the quasi-one-dimensional continuity equation:

(c). 3.2

Similar to a pipetting effect, the negative excess pressure is assumed proportional to the change of the apparent cross-sectional area:

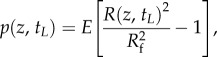(c). 3.3

where Rf is the fully recovered tube radius and E is the apparent area modulus of the tube. Both p and E influence the characteristic time and strength of the elastic recovery. Combining the above momentum, continuity and the elastic equations leads to a nonlinear diffusive pressure equation [16]:

(c). 3.4

originally proposed to simulate a collapsible blood vessel and other physiological scenarios [17–19]. The elasticity of, for example, blood vessels determines the degree of compliance of the tube wall due to the pressure drop; while in the tongue of hummingbirds, the elasticity of the groove walls plays an active pumping role during the recovery of the collapsed configuration. Accordingly, the characteristic length and diffusive time scale are L and 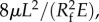Inline graphic respectively. Here, we define an initial condition for the negative excess pressure p (z, 0) = −pa, where pa is a positive constant representing the adhesive energy provided by the thin liquid film remaining in the collapsed tube. It is assumed that the liquid film inside the tongue can temporarily sustain the negative pressure (tension) without cavitation. The boundary condition at the inlet (z = 0) has zero gauge pressure, while the pressure gradient vanishes at the sealed end (z = L). The diffusive pressure field is featured by the relative contributions of negative excess pressure and area modulus, essentially the scaled initial condition, −pa/E. This is the only dimensionless parameter that we use to estimate the self-similar empirical data at various tube lengths.

At the very beginning of the process, because local acceleration is important when the fluid is suddenly drawn into the tube while convective acceleration may be negligible owing to the almost fully developed condition, the above steady approximation is no longer valid in this short-time regime. The elastic relaxation is considered fast and the motion of the tube boundary is much slower than the accelerated momentum transport. We therefore decompose the velocity field for the whole process into transient vz (r, z, t) and quasi-steady ṽz (r, z, tL) contributions, which correspond to the short- and long-time processes, respectively. Substituting the transient and a quasi-steady velocity components, vz + ṽz, respectively, into the linear momentum equation and subtracting the long-time quasi-steady equation by assuming that the pressure effect primarily balances the long-time viscous effect, that is, 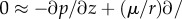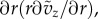Inline graphic Inline graphic the quasi-linear transient flow in the short-time regime can thus be simplified and expressed by the diffusive momentum equation

(c). 3.5

in which the pressure effect vanishes due to the balance with the long-time viscous effect, and the radial velocity contribution compared to the local acceleration, estimated by the variation of tube radius ΔR/Rf, is neglected to facilitate the analytical solution. This approximation is compatible with the quasi-steady boundary condition for the radial motion of the wall. Note that the trial solution of a transient pipe flow (e.g. [16]) implies that the pressure gradient essentially remains time-independent from the transient to quasi-steady processes, and thus for equation (3.5) there is no need to decompose the pressure to short- and long-time contributions. The initial condition is vz (r, z, 0) = − ṽz (r, z, 0). It is expected that the pressure gradient does not play a role in the first approximation of the local acceleration in terms of the transient velocity field vz, and the time dependency of the pressure field only comes from the deformation of the boundary. The boundary conditions are finite velocity vz (0, z, t) along the axial line and the no-slip condition at the wall vz (R(z, tL), z, t) = 0. The analytical solution for the transient velocity field can be expressed as

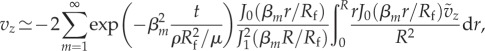(c). 3.6

where J0 and J1 are the zeroth and first-order Bessel functions, respectively, and βm are the eigenvalues given by the no-slip condition J0(βmR/Rf) = 0. As a correction for the leading-order approximation, the initial condition in the integral term here is given by the long-time velocity from the pressure equation

(c). 3.7

Finally, the complete velocity is the summation of both transient and quasi-steady components, vz + ṽz. The local flow rate can also be obtained from the apparent radius and pressure gradient. Equation (3.7) is obtained by direct integration of the Stokes equation twice with respect to r, which preserves the time dependency that comes from the deformation of the boundary. In this case, the nonlinear pressure equation is solved numerically before substituting into the integral solution for the velocity field. Once the velocity of the progressive moving front of the liquid column relative to the tongue is computed, the travelling distance or the filling length can be tracked by a simple Lagrangian integration. Note that the linear approximation here is aimed to accommodate the essential multi-scale behaviour analytically. Perturbative or numerical computation of the full momentum equation, specifically the nonlinear radial velocity contribution in the short-time regime, would be helpful to justify this approximation.

(d). Theoretical versus experimental data

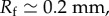
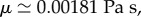
In order to evaluate our model, we compared the model output against our empirical results from actual feeding birds, using the following model parameters: 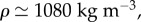Inline graphic Inline graphic Inline graphic pa = 3.3 kPa, E = 4 kPa, and four tube lengths from 10 to 13.4 mm based on experimental measurements. The self-similar dynamics is featured by the ratio pa/E, in which pa controls the pump strength or the peak value of front velocity, while E adjusts the filling time (figure 3). The ratio and both parameters are our best-effort selections based on sensitivity tests (e.g. figure 4) applied to all datasets. The short time scale is on the order of 1 ms, while the long time scale is about 10–20 ms. The Reynolds number is up to an order of 100 at the peak velocity. The results show a sub-linear increase of the observable front velocity (figure 3a) and a super-linear increase of the filling length (equivalent to the front position h(t), figure 3b). The filling length ∝ tn and filling velocity ∝ tn−1, where 1.0 < n < 1.5, indicate that the inertial force on the fluid drawn from the nectar pool is more significant than the result obtained from the inertia-dominated capillarity rise model [21], where n = 1, and of course than the quasi-steady case, where n = 0.5 based on the Lucas–Washburn model [22]. The duration for the transition from super-linear to sub-linear increase of the filling length is relatively long compared with the short time scale; this is perhaps why others have been led to believe that capillarity (n = 1) is the primary driving mechanism. This is understandable because capillarity, regardless of the contact angle, provides a pulling force very similar to a pressure drop across the liquid column. However, comparing with the Bosanquet's capillary model [20] that takes inertial effect into account (figure 3b), even under the largest effective surface tension force, based on contact angle of zero, the capillarity model cannot match our fast empirical filling data. Note that Bosanquet's capillary model considers the balance of pressure, capillarity, inertial and viscous effects. 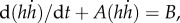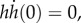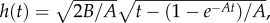The transient equation, initial conditions and the solution for the capillary rise h(t) can be expressed as Inline graphic h(0) = 0 and Inline graphic and Inline graphic respectively, where A = 8μ/ρR2 and B = (2γcosθ/R + p0)/ρ, and all parameters involved follow their typical definitions. The gravity effect is neglected in Bosanquet's analytical result.

Figure 3.

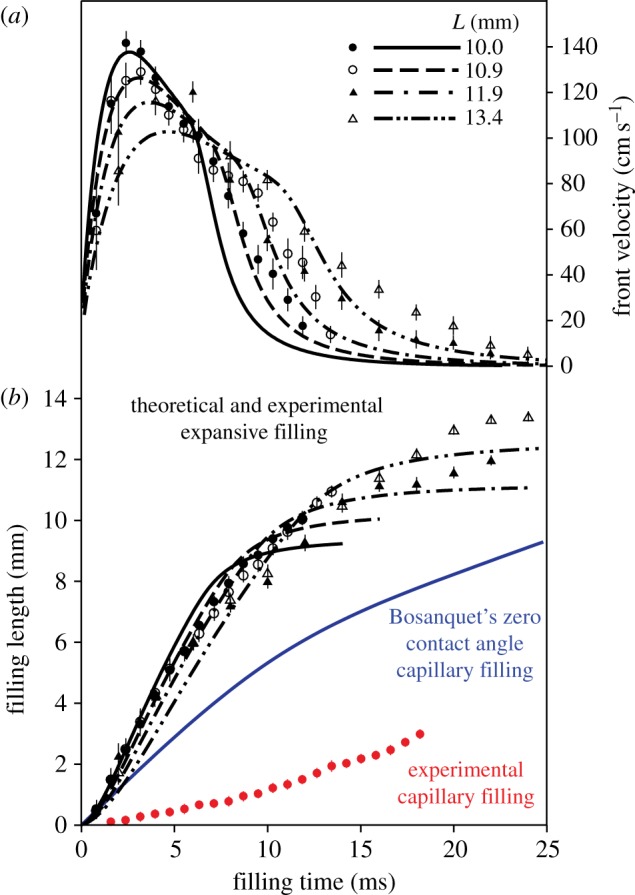
Figure 3.

Open in a new tab

Theoretical and experimental data for the filling mechanisms in hummingbird tongues. (a) Transient velocity of the travelling liquid front versus time. Data points (black symbols) denote expansive filling data from four drinking sequences of free-living birds, exemplifying different filling tube lengths (mm). Lines (black) are predictions from our elastohydrodynamic model. (b) Transient filling length h versus time at the same tube lengths shown above. Bosanquet's [20] capillary model curve corresponds to theoretical capillary filling, that takes inertial effect into consideration, under a zero contact angle condition (complete wetting). All parameters used in Bosanquet's model, including fluid properties and relaxed tube size, follow the expansive filling model except that the length of the tube is irrelevant for Bosanquet's result. Experimental capillary filling data points (circles at the bottom) represent our only observation of capillarity, from only one side of the tongue, in a single lick (electronic supplementary material, video S3). Vertical bars correspond to 95% confidence intervals (CIs) from five repeated measurements of each filling event. (Online version in colour.)

Figure 4.

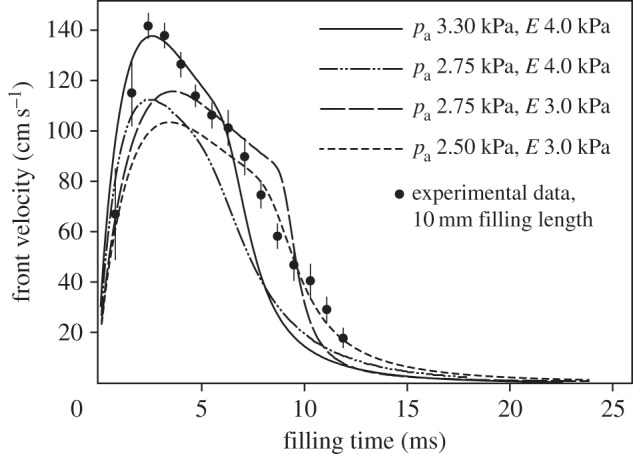
Figure 4.

Open in a new tab

Sensitivity tests for the elastohydrodynamic model of the filling mechanism in hummingbird tongues. The graph represents the comparison of calculated front velocity versus time based on various negative excess pressure −pa and area modulus E. The lines are predictions from our elastohydrodynamic model, and the data points are experimental results for L = 10 mm. Vertical bars correspond to 95% CIs from five repeated measurements.

In hundreds of licks studied, we observed capillarity only once and acting in only a single tongue groove (electronic supplementary material, video S3). In this event, at the initial stages of tongue protrusion, one of the groove tips adhered to the feeder wall before the tip reached the surface of the nectar pool, and the stuck groove tip bent as the tongue continued to move forward. We surmise that the bending caused the groove to lose its collapsed configuration and recover its cylindrical, empty, shape when still outside the nectar. Before the tongue contacted the liquid surface, the groove tip slid forward and entered the nectar unbent. Thanks to this unusual accident, we captured one of the two grooves being filled by expansive filling (as usual), while the other was being filled by capillarity, offering a fortuitous opportunity to directly compare the two mechanisms. Our measured capillary filling rate (circles at the bottom, figure 3b) is an order of magnitude slower than expansive filling when all other factors are equal; therefore, capillarity cannot be the nectar uptake mechanism observed in all the other licks.

Figure 3a shows the front velocity at r = 0 and z = h(t); in the short time regime, the elastic force overcomes the inertial effect to pull the nectar into the tube, while at the long time regime, the elasticity-induced negative excess pressure balances the fluid viscous effect. The first transition appears at the peak velocity on the order of 1 m s−1, and then decays as the diffusive pressure wave travels along the tube length (figure 2c). The second transition that is evident in the modelling results is when the pressure wave reaches the sealed end of the tube, and the tube profiles diffusively relax to an equilibrium shape. Considering the case of L = 10 mm, at the decaying stage, the velocity changes its slope at around 6–7 ms. Because the characteristic time scale τ = 8μL2/(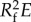Inline graphic) ≈ 9 ms, the profile shown in figure 2c at 0.7τ ≈ 6–7 ms explains that such a transition appears when the dynamic cross-sectional tube profiles transit from wave-like to diffusive-like behaviours. The various capillarity models that have been applied to hummingbird feeding are not able to describe the observed short- to long-time behaviours and the interesting dynamics at both transitions.

The fit of output from our first-order-only approximation to our empirical measurements (figure 3) is satisfactory, and provides support for our conceptual explanation of the micropumping mechanism. Figure 4 shows one of the sensitivity tests we performed using various negative excess pressure −pa and area modulus E values. Overall, a larger excess pressure results in higher peak velocity, while the time scale or width of the profile is more affected when the E value is modified. Note that the selection of the parameters pa and E is not arbitrary. As mentioned earlier, the self-similar dynamics is featured by the relative contributions of negative excess pressure and area modulus −pa/E as the only characteristic number in this model. Our best fit of pa/E is about 0.83, corresponding to two cases: pa = 3.3, E = 4.0 that better predicts the peak value of the front velocity, and pa = 2.5, E = 3.0 that fit better to the long-time profile. Another two cases with smaller or larger pa/E value, about 0.69 and 0.92 are not good selections. We thus choose pa = 3.3, E = 4.0 for all cases in figure 3 to emphasize the maximum front velocity. The prediction for the long-time behaviour may be improved by gradually reducing the thickness of the tube towards the end (taking into account that hummingbird tongue grooves taper off towards the base).

4. Discussion

Our dataset of direct measurements of tongue/nectar interactions during nectar uptake in free-living wild birds refutes the century-old paradigm that posits capillary rise as the main mechanism operating during hummingbird drinking. Capillary rise is physically possible in hummingbird tongues, but, in the wild, it simply is not biologically relevant.

Combining the elastohydrodynamic model we present here with our previous work on the mechanics of fluid trapping at the tongue tips [14], we now have an empirically supported, mechanistic understanding of nectar intake in hummingbirds with which to newly interpret their energetics and ecology. It is noteworthy that at flowers where full groove immersion is prohibited by corolla length, filling lengths are usually equivalent to the distances between the bill tip and the nectar surface. Given that it is only at great filling lengths (when the bill tip is far from the nectar surface and full groove immersion is precluded) that a tongue-filling mechanism besides nectar trapping at the groove tips [14] would contribute a significant portion of the total load per lick, we rule out capillarity as an important drinking mechanism in free-living birds feeding at real flowers.

The average flow rate, a measure of interest for ecology and engineering applications, would be calculated as the volume of liquid collected per unit time. Given that the end result of both capillarity and expansive filling is a tongue groove entirely filled with nectar, the volume would be exactly the same under the two scenarios. However, a groove would be filled appreciably slower via capillarity (e.g. figure 3; electronic supplementary material, video S3). The slow fill speed of capillarity would therefore limit the hummingbird's licking rate, and thus their energy intake rate. This is manifest in the time interval for the one full lick cycle reported elsewhere as the result of capillarity [8]: 200 ms, which translates into a licking rate of only 5 Hz. This is very low when compared to the licking rates registered for larger samples of birds, under more realistic conditions, which are around 14 Hz [23]. If the tongue normally worked via capillarity in free-living hummingbirds, we would not observe the high licking rates that have been reported (up to 17 Hz [15]). On the other hand, the elastic micropump we describe here allows for tongue loading at rates that are compatible with previously reported licking rates. Thus, we conclude that the capillary tongue-filling reported in laboratory studies (e.g. [8]) is the result of feeding under unnatural conditions, and therefore is not representative of the processes operating in wild birds under biologically relevant conditions.

Fluid trapping is the predominant process by which hummingbirds achieve nectar collection at small bill tip-to-nectar distances, wherein tongue grooves are wholly immersed in nectar, or when the nectar is found in very thin layers [14]. Expansive filling accounts for nectar uptake by the portions of a hummingbird's tongue that remain outside the nectar pool. The relative contributions of the two synergistic mechanisms (fluid trapping and expansive filling) to the rate and volume of nectar ultimately ingested are determined by the distance from the bill tip to the nectar surface during the licking process. Updating feeding efficiency estimates in light of these advances could provide new insights critical to current evolutionary debates (e.g. optimal nectar concentrations [9,11,24,25]). In addition it could also improve our understanding of broad-scale ecological patterns (e.g. species range limits and competition in hummingbird assemblages [6], and phenological shifts with conservation implications [26]).

A reappraisal of preference experiments along gradients of nectar concentrations [27–32] using these new nectar intake models and applying recent advances on gustatory discrimination [33], could shed new light on coevolutionary enigmas. Several plant lineages have converged on a transition from an insect-pollinated condition to vertebrate-pollination [34–37], and vertebrate-pollinated flowers tend to have more dilute nectars than the insect-pollinated ones [24,38–40]. The new explanation of the mechanics of nectar uptake we provide here suggests that physical constraints are the main determinants of the relationship between pollinator type and nectar concentration, and can guide us through alternative hypotheses [40] of hummingbird–flower coevolution. Our discovery of this elastic tongue micropump could inspire applications, and the study of flow, in elastic-walled (flexible) tubes in both biological [41,42] and artificial [43–46] systems.

5. Methods

(a). Fieldwork

At field sites with existing feeders in seven countries throughout the Americas, we filmed free-living, never handled, hummingbirds drinking at modified transparent feeders simulating the nectar volumes and concentrations of hummingbird-pollinated flowers. We measured 96 foraging bouts of 32 focal birds belonging to 18 species from seven out of the nine main hummingbird clades (electronic supplementary material, table S1). We used artificial nectar (18.6% mass/mass sucrose concentration) and focused on recording the tongue–fluid interaction using high-speed cameras (TroubleShooter HR and Phantom Miro ex4) with macro lenses (Nikon 105 mm f/2.8 VR) running up to 1260 frames per second (1280 × 512 pixels). We positioned red flat plastic sheets (to minimize lateral view obstruction) cut in flower shapes at the entrances of the feeders. The purpose of the flat flowers was two-fold: to attract and guide wild hummingbirds into our feeders, and to allow us to control the relative position of the artificial corolla entrance with respect to the nectar chamber. While filming wild hummingbirds, we noted that every individual, after a couple of exploratory visits, would insert its beak as far as possible into the feeder in order to reach the nectar. At real flowers, corolla length limits how close the bird can place the tip of its beak to the surface of the nectar pool. Controlling the position of the flat flower with respect to the nectar reservoir, we achieved videos in which there is enough realistic distance between the bill tip and the nectar surface to study the filling of the tongue portions that never enter the liquid (e.g. electronic supplementary material, video S4). To improve visualization of the filling front, while filming Amazilia hummingbirds (Amazilia amazilia) in Ecuador, we used hummingbird nectar concentrate (Petco®), which comes tinted red, and we diluted it (down to 18.6% mass/mass concentration).

(b). Velocity and thickness measurements

We limited our measurements to the instances in which we could confidently track the tongue tip and the groove bases throughout the entire lick. We calculated tongue tip velocity through time and estimated fluid displacement velocities using ImageJ [47]. To measure groove thickness at regular length intervals, we delineated the contour of the tongue in tpsDig 2.16 [48]. Subsequently, we limited these outlines between the tip and the base of the grooves and resampled dorsal and ventral outlines into 20 semi-landmarks [49,50]. These semi-landmarks allowed us to calculate groove thickness at equally spaced points along the tongue through the licking cycle, and to quantitatively test predictions from the mutually exclusive possible outcomes. We tested whether there was a difference in groove thickness before and after contact with the nectar (complete filling), using a two-tailed Student's paired t-test, after testing for normality through a Shapiro–Wilk test. Having good estimates of the position of groove tip and base is important to provide accurate measurements of the groove thickness at comparable points (semilandmarks) across time, licks, individuals and species. For these comparative measurements, we used the semi-landmark no. 12 (near the middle of the grooves) and calculated the percentage, out of the final thickness of the groove, that the expansion represents (electronic supplementary material, table S1).

Supplementary Material

Table S1. Expansion of the tongue in 32 hummingbirds of 18 species.
rspb20151014supp1.pdf (4.7MB, pdf)

Acknowledgements

We thank D. Sustaita, C. Elphick, R. Colwell, K. Schwenk, C. Field, K. Hurme, E. Hurme, H. Brown and the UConn Ornithological Research Group for feedback. We are grateful to C. Clark, and D. Altshuler for the loan of high-speed cameras and logistic support. Finally, we thank L. Rico, A. Morales, L. Cárdenas and O. Acevedo for fieldwork assistance.

Ethics

All filming activities were reviewed and authorized by the Institutional Animal Care and Use Committee at the University of Connecticut; Exemption Number E09-010.

Authors' contributions

A.R.-G. and M.A.R. conceived the project and designed the experiments. T.-H.F. performed the fluid dynamics simulations and mathematical modelling. A.R.-G. conducted the experiments and analysed the data. All authors discussed results and commented on manuscript.

Competing interests

We declare we have no competing interests.

Funding

A.R.-G. was funded by: UConn EEB Department, CESE, American Ornithologists’ Union, Sigma-Xi and NSF IOS- DDIG 1311443, and T.-H.F. acknowledges the support from NSF CMMI-0952646.

References

  • 1.Snow BK, Snow DW. 1972. Feeding niches of hummingbirds in a Trinidad valley. J. Anim. Ecol. 41, 471–485. ( 10.2307/3481) [DOI] [Google Scholar]
  • 2.Feinsinger P, Colwell RK. 1978. Community organization among Neotropical nectar-feeding birds. Am. Zool. 18, 779–795. ( 10.1093/icb/18.4.779) [DOI] [Google Scholar]
  • 3.Brown JH, Bowers MA. 1985. Community organization in hummingbirds: relationships between morphology and ecology. Auk 102, 251–269. ( 10.2307/4086767) [DOI] [Google Scholar]
  • 4.Stiles FG. 1985. Seasonal patterns and coevolution in the hummingbird-flower community of a Costa Rican subtropical forest. Ornithol. Monogr. 36, 757–787. ( 10.2307/40168315) [DOI] [Google Scholar]
  • 5.Rahbek C, Graves GR. 2000. Detection of macro-ecological patterns in South American hummingbirds is affected by spatial scale. Proc. R. Soc. Lond. B 267, 2259–2265. ( 10.1098/rspb.2000.1277) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 6.Vizentin-Bugoni J, Maruyama PK, Sazima M. 2014. Processes entangling interactions in communities: forbidden links are more important than abundance in a hummingbird–plant network. Proc. R. Soc. B 281, 20132397 ( 10.1098/rspb.2013.2397) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 7.Martin WCL. 1833. The naturalist's library: a general history of humming-birds or the Trochilidae, vol. 41 (ed. Jardine W.), pp. 65–68. London, UK: H.G. Bohn. [Google Scholar]
  • 8.Kim W, Peaudecerf F, Baldwin MW, Bush JWM. 2012. The hummingbird's tongue: a self-assembling capillary syphon. Proc. R. Soc. B 279, 4990–4996. ( 10.1098/rspb.2012.1837) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 9.Kim W, Gilet TT, Bush JWM. 2011. Optimal concentrations in nectar feeding. Proc. Natl Acad. Sci. USA 108, 16 618–16 621. ( 10.1073/pnas.1108642108) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 10.Kingsolver JG, Daniel TL. 1983. Mechanical determinants of nectar feeding strategy in hummingbirds: energetics, tongue morphology, and licking behavior. Oecologia 60, 214–226. ( 10.1007/BF00379523) [DOI] [PubMed] [Google Scholar]
  • 11.Heyneman AJ. 1983. Optimal sugar concentrations of floral nectars: dependence on sugar intake efficiency and foraging costs. Oecologia 60, 198–213. ( 10.1007/BF00379522) [DOI] [PubMed] [Google Scholar]
  • 12.Kim W, Bush JWM. 2012. Natural drinking strategies. J. Fluid Mech. 705, 7–25. ( 10.1017/jfm.2012.122) [DOI] [Google Scholar]
  • 13.Jensen KH, Kim W, Holbrook NM, Bush JWM. 2012. Optimal concentrations in transport systems. J. R. Soc. Interface 10, 20130138 ( 10.1098/rsif.2013.0138) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 14.Rico-Guevara A, Rubega MA. 2011. The hummingbird tongue is a fluid trap, not a capillary tube. Proc. Natl Acad. Sci. USA 108, 9356–9360. ( 10.1073/pnas.1016944108) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 15.Ewald PW, Williams WA. 1982. Function of the bill and tongue in nectar uptake by hummingbirds. Auk 99, 573–576. [Google Scholar]
  • 16.Middleman S. 1997. An introduction to fluid dynamics: principles of analysis and design. New York, NY: Wiley. [Google Scholar]
  • 17.Womersley JR. 1955. Method for the calculation of velocity, rate of flow and viscous drag in arteries when the pressure gradient is known. J. Physiol. 127, 553–563. ( 10.1113/jphysiol.1955.sp005276) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 18.Katz AI, Chen Y, Moreno AH. 1969. Flow through a collapsible tube. Biophys. J. 9, 1261–1279. ( 10.1016/S0006-3495(69)86451-9) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 19.Rubinow SI, Keller JB. 1972. Flow of a viscous fluid through an elastic tube with applications to blood flow. J. Theor. Biol. 35, 299–313. ( 10.1016/0022-5193(72)90041-0) [DOI] [PubMed] [Google Scholar]
  • 20.Bosanquet CH. 1923. LV. On the flow of liquids into capillary tubes. Phil. Mag. 45, 525–531. ( 10.1080/14786442308634144) [DOI] [Google Scholar]
  • 21.Quéré D. 1997. Inertial capillarity. Europhys. Lett. 39, 533–538. ( 10.1209/epl/i1997-00389-2) [DOI] [Google Scholar]
  • 22.Zhmud BV, Tibert F, Hallstensson K. 2000. Dynamics of capillary rise. J. Colloid Interface Sci. 228, 263–269. ( 10.1006/jcis.2000.6951) [DOI] [PubMed] [Google Scholar]
  • 23.Rico-Guevara A, Rubega MA. 2012. Hummingbird feeding mechanics: comments on the capillarity model. Proc. Natl Acad. Sci. USA 109, E867 ( 10.1073/pnas.1119750109) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 24.Pyke GH, Waser NM. 1981. The production of dilute nectars by hummingbird and honeyeater flowers. Biotropica 13, 260–270. ( 10.2307/2387804) [DOI] [Google Scholar]
  • 25.Johnson SD, Nicolson SW. 2008. Evolutionary associations between nectar properties and specificity in bird pollination systems. Biol. Lett. 4, 49–52. ( 10.1098/rsbl.2007.0496) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 26.McKinney AMA, CaraDonna PJ, Inouye DW, Barr BB, Bertelsen CDC, Waser NMN. 2012. Asynchronous changes in phenology of migrating broad-tailed hummingbirds and their early-season nectar resources. Ecology 93, 1987–1993. ( 10.1890/12-0255.1) [DOI] [PubMed] [Google Scholar]
  • 27.Hainsworth FR, Wolf LL. 1976. Nectar characteristics and food selection by hummingbirds. Oecologia 25, 101–113. ( 10.1007/BF00368847) [DOI] [PubMed] [Google Scholar]
  • 28.Stiles FG. 1976. Taste preferences, color preferences, and flower choice in hummingbirds. Condor 78, 10–26. ( 10.2307/1366912) [DOI] [Google Scholar]
  • 29.Tamm S, Gass CL. 1986. Energy intake rates and nectar concentration preferences by hummingbirds. Oecologia 70, 20–23. ( 10.1007/BF00377107) [DOI] [PubMed] [Google Scholar]
  • 30.Roberts WM. 1996. Hummingbirds’ nectar concentration preferences at low volume: the importance of time scale. Anim. Behav. 52, 361–370. ( 10.1006/anbe.1996.0180) [DOI] [Google Scholar]
  • 31.Schondube JE, Martínez-Del Rio C. 2003. Concentration-dependent sugar preferences in nectar-feeding birds: mechanisms and consequences. Funct. Ecol. 17, 445–453. ( 10.1046/j.1365-2435.2003.00749.x) [DOI] [Google Scholar]
  • 32.Guzman WA, Wilson P. 2012. Hummingbirds at artificial flowers made to resemble ornithophiles versus melittophiles. J. Poll. Ecol. 8, 67–78. [Google Scholar]
  • 33.Nachev V, Stich KP, Winter Y. 2013. Weber's law, the magnitude effect and discrimination of sugar concentrations in nectar-feeding animals. PLoS ONE 8, e74144 ( 10.1371/journal.pone.0074144) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 34.Thomson JD, Wilson P. 2008. Explaining evolutionary shifts between bee and hummingbird pollination: convergence, divergence, and directionality. Int. J. Plant Sci. 169, 23–38. ( 10.1086/523361) [DOI] [Google Scholar]
  • 35.Fleming T, Geiselman C, Kress W. 2009. The evolution of bat pollination: a phylogenetic perspective. Ann. Bot. 104, 1017–1043. ( 10.1093/aob/mcp197) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 36.van der Niet T, Johnson SD. 2012. Phylogenetic evidence for pollinator-driven diversification of angiosperms. Trends Ecol. Evol. 27, 353–361. ( 10.1016/j.tree.2012.02.002) [DOI] [PubMed] [Google Scholar]
  • 37.Barrett SCH. 2013. The evolution of plant reproductive systems: how often are transitions irreversible? Proc. R Soc. B 280, 20130913 ( 10.1098/rspb.2013.0913) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 38.Baker HG. 1975. Sugar concentrations in nectars from hummingbird flowers. Biotropica 7, 37–41. ( 10.2307/2989798) [DOI] [Google Scholar]
  • 39.Nicolson SW. 2002. Pollination by passerine birds: why are the nectars so dilute? Comp. Biochem. Physiol. B 131, 645–652. ( 10.1016/S1096-4959(02)00014-3) [DOI] [PubMed] [Google Scholar]
  • 40.Nicolson SW, Fleming PA. 2003. Nectar as food for birds: the physiological consequences of drinking dilute sugar solutions. Plant Syst. Evol. 238, 139–153. [Google Scholar]
  • 41.Grotberg JB, Jensen OE. 2004. Biofluid mechanics in flexible tubes. Annu. Rev. Fluid Mech. 36, 121–147. ( 10.1146/annurev.fluid.36.050802.121918) [DOI] [Google Scholar]
  • 42.Hazel AL, Heil M. 2005. Surface-tension-induced buckling of liquid-lined elastic tubes: a model for pulmonary airway closure. Proc. R Soc. A 461, 1847–1868. ( 10.1098/rspa.2005.1453) [DOI] [Google Scholar]
  • 43.Sun D, Shu D, Ji M, Liu F, Wang M, Gong X. 2004. Pressure-induced hard-to-soft transition of a single carbon nanotube. Phys. Rev. B Condens. Matter 70, 165417 ( 10.1103/PhysRevB.70.165417) [DOI] [Google Scholar]
  • 44.Marzo A, Luo XY, Bertram CD. 2005. Three-dimensional collapse and steady flow in thick-walled flexible tubes. J. Fluid Struct. 20, 817–835. ( 10.1016/j.jfluidstructs.2005.03.008) [DOI] [Google Scholar]
  • 45.Nahar S, Jeelani SAK, Windhab EJ. 2012. Influence of elastic tube deformation on flow behavior of a shear thinning fluid. Chem. Eng. Sci. 75, 445–455. ( 10.1016/j.ces.2012.03.051) [DOI] [Google Scholar]
  • 46.Nahar S, Jeelani SAK, Windhab EJ. 2013. Prediction of velocity profiles of shear thinning fluids flowing in elastic tubes. Chem. Eng. Commun. 200, 820–835. ( 10.1080/00986445.2012.722150) [DOI] [Google Scholar]
  • 47.Schneider CA, Rasband WS, Eliceiri KW. 2012. NIH Image to ImageJ: 25 years of image analysis. Nat. Methods 9, 671–675. ( 10.1038/nmeth.2089) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 48.Rohlf FJ. 2010. TPSDig2 version 2.16. New York, NY: Stony Brook University. [Google Scholar]
  • 49.Bookstein FL. 1991. Morphometric tools for landmark data: geometry and biology. Cambridge, UK: Cambridge University Press. [Google Scholar]
  • 50.Mitteroecker P, Gunz P. 2009. Advances in geometric morphometrics. Evol. Biol. 36, 235–247. ( 10.1007/s11692-009-9055-x) [DOI] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Table S1. Expansion of the tongue in 32 hummingbirds of 18 species.
rspb20151014supp1.pdf (4.7MB, pdf)

Articles from Proceedings of the Royal Society B: Biological Sciences are provided here courtesy of The Royal Society

RESOURCES