Abstract
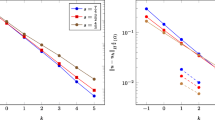
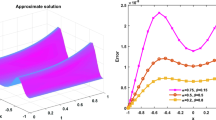
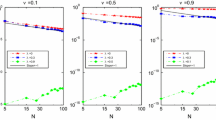
In this paper, we develop an h-p version of finite element method for one-dimensional fractional differential equation \(-_{0}D_{x}^{\alpha }u+Au=f(x)\) with Dirichlet boundary condition. First, we introduce the existence and uniqueness of the considered problem and show the wellposedness of the corresponding weak form. To solve the mentioned equation, the classical hierarchical polynomials are employed as the trial basis functions. Then, we develop a kind of novel test basis functions for the Petrov-Galerkin finite element method such that the stiffness matrix becomes an identity matrix and the coefficient matrix often has a small condition number. Moreover, we give some properties of the developed test basis functions, and discuss the implementation of the developed finite element method in detail. It is shown that the implementation of our method is easier than that of other finite (and spectral) element methods. Finally, we give a numerical example, and the numerical results show the effectiveness of the develped method.
Similar content being viewed by others
References
Castillo-Negrete, D., del Carreras, B., Lynch, V.: Front dynamics in reaction-diffusion systems with levy flights: a fractional diffusion approach. Phys. Rev. Lett. 91, 018302 (2003)
Chen, M., Deng, W.: Fourth order accurate scheme for the space fractional diffusion equations. SIAM J. Numer. Anal. 52, 1418–1438 (2014)
Ding, Z., Xiao, A., Li, M.: Weighted finite difference methods for a class of space fractional partial differential equations with variable coefficients. J. Comput. Appl. Math. 233, 1905–1914 (2010)
Ervin, V.J., Roop, J.P.: Variational formulation for the stationary fractional advection dispersion equation. Numer. Meth. Part. D. E 22, 558–576 (2006)
Herrmann, R.: Fractional Calculus: an Introduction for Physicists. World Scientific, Singapore (2011)
Hilfer, R.: Applications of Fractional Calculus in Physics. World Scientific, New Jersey (2000)
Jin, B., Lazarov, R., Pasciak, J., Rundell, W.: Variational formulation of problems involving fractional order differential operators. Math. Comp. 84, 2665–2700 (2015)
Jin, B., Lazarov, R., Zhou, Z.: A Petrov–Galerkin finite element method for fractional convection-diffusion equations. SIAM J. Numer. Anal. 54, 481–503 (2016)
Kharazmi, E., Zayernouri, M., Karniadakis, G.E.: A Petrov–Galerkin spectral element method for fractional elliptic problems. Comput. Methods Appl. Mech. Engrg. 324, 512–536 (2017)
Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications Of Fractional Differential Equations. Elsevier, Boston (2006)
Kopteva, N., Stynes, M.: Analysis and numerical solution of a Riemann-Liouville fractional derivative two-point boundary value problem. Adv. Comput. Math. 43, 77–99 (2017)
Liu, F., Zhuang, P., Anh, V., Turner, I., Burrage, K.: Stability and convergence of the difference methods for the space-time fractional advection-diffusion equation. Appl. Math. Comput. 191, 12–20 (2007)
Mao, Z., Shen, J.: Spectral element method with geometric mesh for two-sided fractional differential equations. Adv. Comput. Math. https://doi.org/10.1007/s10444-017-9561-9 (2017)
Meerschaert, M.M., Tadjeran, C.: Finite difference approximations for two-sided space-fractional partial differential equations. Appl. Numer. Math. 56, 80–90 (2006)
Metzler, R., Klafter, J.: The random walk’s guide to anomalous diffusion: a fractional dynamics approach. Phys. Rep. 339, 1–77 (2000)
Podlubny, I.: Fractional Differential Equations. Academic Press, New York (1999)
Shen, J., Tang, T., Wang, L.: Spectral Methods: Algorithms, Analysis and Applications. Springer, Berlin (2011)
Sousa, E.: A second order explicit finite difference method for the fractional advection diffusion equation. Comput. Math. Appl. 64, 3141–3152 (2012)
Stynes, M., O’riordan, E., Gracia, J.L.: Error analysis of a finite difference method on graded meshes for a time-fractional diffusion equation. SIAM J. Numer. Anal. 55, 1057–1079 (2017)
Tadjeran, C., Meerschaert, M.M., Scheffler, H.-P.: A second-order accurate numerical approximation for the fractional diffusion equation. J. Comput. Phys. 213, 205–213 (2006)
Wang, H., Yang, D., Zhu, S.: Inhomogeneous Dirichlet boundary-value problems of space-fractional diffusion equations and their finite element approximations. SIAM J. Numer. Anal. 52, 1292–1310 (2014)
Zayernouri, M., Karniadakis, G.E.: Fractional Sturm–Liouville eigen-problems: theory and numerical approximation. J. Comput. Phys. 252, 495–517 (2013)
Zhao, Y., Bu, W., Zhao, X., Tang, Y.: Galerkin finite element method for two-dimensional space and time fractional Bloch–Torrey equation. J. Comput. Phys. 350, 117–135 (2017)
Zheng, Y., Li, C., Zhao, Z.: A note on the finite element method for the space-fractional advection diffusion equation. Comput. Math. Appl. 59, 1718–1726 (2010)
Acknowledgements
The first author sincerely thanks Prof. George Em Karniadakis for giving a chance to visit Brown University for two months.
Funding
This research is supported by the National Natural Science Foundation of China (Nos. 11601460, 11671343), the Natural Science Foundation of Hunan Province of China (No. 2018JJ3491), and the Research Foundation of Education Commission of Hunan Province of China (No. 16C1540).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Bu, W., Xiao, A. An h-p version of the continuous Petrov-Galerkin finite element method for Riemann-Liouville fractional differential equation with novel test basis functions. Numer Algor 81, 529–545 (2019). https://doi.org/10.1007/s11075-018-0559-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11075-018-0559-2