iBet uBet web content aggregator. Adding the entire web to your favor.
iBet uBet web content aggregator. Adding the entire web to your favor.



Link to original content: https://unpaywall.org/10.1007/978-3-642-33860-1_12
On the Security of Interferometric Quantum Key Distribution | SpringerLink
Skip to main content
Springer Nature Link
Log in

On the Security of Interferometric Quantum Key Distribution

  • Conference paper
Theory and Practice of Natural Computing (TPNC 2012)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 7505))

Included in the following conference series:

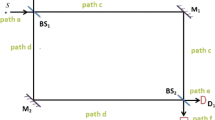
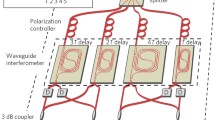
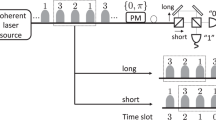
Abstract

Photonic quantum key distribution (QKD) is commonly implemented using interferometers, devices that inherently cause the addition of vacuum ancillas, thus enlarging the quantum space in use. This enlargement sometimes exposes the implemented protocol to new kinds of attacks that have not yet been analyzed.

We consider several QKD implementations that use interferometers, and analyze the enlargement of the quantum space caused by the interferometers. While we show that some interferometric implementations are robust (against simple attacks), our main finding is that several other implementations used in QKD experiments are totally insecure.

This result is somewhat surprising since although we assume ideal devices and an underlying protocol which is proven secure (e.g., the Bennett-Brassard QKD), the realization is insecure. Our novel attack demonstrates the risks of using practical realizations without performing an extensive security analysis of the specific setup in use.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
USD 54.99
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
USD 72.00
Price excludes VAT (USA)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Aharonov, Y., Bergmann, P.G., Lebowitz, J.L.: Time symmetry in the quantum process of measurement. Phys. Rev. 134(6B), B1410–B1416 (1964)

    Article  MathSciNet  Google Scholar 

  2. Aharonov, Y., Vaidman, L.: Properties of a quantum system during the time interval between two measurements. Physical Review A 41(1), 11–20 (1990)

    Article  MathSciNet  Google Scholar 

  3. Ben-Or, M., Horodecki, M., Leung, D.W., Mayers, D., Oppenheim, J.: The Universal Composable Security of Quantum Key Distribution. In: Kilian, J. (ed.) TCC 2005. LNCS, vol. 3378, pp. 386–406. Springer, Heidelberg (2005)

    Chapter  Google Scholar 

  4. Bennett, C.H.: Quantum cryptography using any two nonorthogonal states. Physical Review Letters 68(21), 3121–3124 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bennett, C.H., Brassard, G.: Quantum Cryptography: Public key distribution and coin tossing. In: Proceedings of IEEE International Conference on Computers, Systems and Signal Processing, pp. 175–179 (December 1984)

    Google Scholar 

  6. Biham, E., Boyer, M., Boykin, P.O., Mor, T., Roychowdhury, V.P.: A proof of the security of quantum key distribution. J. Cryptology 19(4), 381–439 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  7. Biham, E., Huttner, B., Mor, T.: Quantum cryptographic network based on quantum memories. Physical Review A 54(4), 2651–2658 (1996)

    Article  MathSciNet  Google Scholar 

  8. Bonfrate, G., Harlow, M., Ford, C., Maxwell, G., Townsend, P.: Asymmetric mach-zehnder germano-silicate channel waveguide interferometers for quantum cryptography systems. Electronics Letters 37(13), 846–847 (2001)

    Article  Google Scholar 

  9. Boyer, M., Kenigsberg, D., Mor, T.: Quantum Key Distribution with Classical Bob. Physical Review Letters 99(14), 140501 (2007)

    Article  MathSciNet  Google Scholar 

  10. Boyer, M., Gelles, R., Kenigsberg, D., Mor, T.: Semiquantum key distribution. Physical Review A 79(3), 032341 (2009)

    Article  MathSciNet  Google Scholar 

  11. Boyer, M., Gelles, R., Mor, T.: Attacks on Fixed Apparatus Quantum Key Distribution Schemes. In: Dediu, A.-H., Martín-Vide, C., Truthe, B. (eds.) TPNC 2012. LNCS, pp. 97–107. Springer, Heidelberg (2012)

    Google Scholar 

  12. Boykin, P.O., Roychowdhury, V.P.: Information vs. disturbance in dimension d. Quantum Info. Comput. 5(4), 396–412 (2005)

    MathSciNet  MATH  Google Scholar 

  13. Brassard, G., Lütkenhaus, N., Mor, T., Sanders, B.C.: Limitations on practical quantum cryptography. Physical Review Letters 85(6), 1330–1333 (2000)

    Article  Google Scholar 

  14. Bruß, D.: Optimal Eavesdropping in Quantum Cryptography with Six States. Physical Review Letters 81, 3018–3021 (1998)

    Article  Google Scholar 

  15. Dusek, M., Lütkenhaus, N., Hendrych, M.: Chapter 5 quantum cryptography. In: Wolf, E. (ed.) Progress in Optics, vol. 49, pp. 381–454. Elsevier (2006)

    Google Scholar 

  16. Elliott, C., Pearson, D., Troxel, G.: Quantum cryptography in practice. In: SIGCOMM 2003, pp. 227–238 (2003)

    Google Scholar 

  17. Fuchs, C.A., Gisin, N., Griffiths, R.B., Niu, C.S., Peres, A.: Optimal eavesdropping in quantum cryptography. i. information bound and optimal strategy. Physical Review A 56(2), 1163–1172 (1997)

    Article  MathSciNet  Google Scholar 

  18. Fuchs, C.A., Peres, A.: Quantum-state disturbance versus information gain: Uncertainty relations for quantum information. Physical Review A 53(4), 2038–2045 (1996)

    Article  Google Scholar 

  19. Gelles, R., Mor, T.: On the security of interferometric quantum key distribution (2011), (full version) arXiv:1110.6573

    Google Scholar 

  20. Gisin, N., Ribordy, G., Tittel, W., Zbinden, H.: Quantum cryptography. Reviews of Modern Physics 74(1), 145–195 (2002)

    Article  Google Scholar 

  21. Gobby, C., Yuan, Z.L., Shields, A.J.: Quantum key distribution over 122 km of standard telecom fiber. Applied Physics Letters 84(19), 3762–3764 (2004)

    Article  Google Scholar 

  22. Gottesman, D., Lo, H.-K., Lütkenhaus, N., Preskill, J.: Security of quantum key distribution with imperfect devices. Quantum Information and Computation 5, 325–360 (2004)

    Google Scholar 

  23. Hughes, R.J., Luther, G.G., Morgan, G.L., Simmons, C.: Quantum cryptography over 14km of installed optical fiber. In: Rochester Conference on Coherence and Quantum Optics, pp. 7–10 (June 1995)

    Google Scholar 

  24. Inamori, H., Lütkenhaus, N., Mayers, D.: Unconditional security of practical quantum key distribution. European Physical Journal D 41, 599–627 (2007)

    Article  Google Scholar 

  25. Inoue, K., Waks, E., Yamamoto, Y.: Differential phase shift quantum key distribution. Physical Review Letters 89(3), 037902 (2002)

    Article  Google Scholar 

  26. Jaeger, G., Sergienko, A.: Entangled states in quantum key distribution. In: AIP Conference Proceedings, vol. 810(1), pp. 161–167 (2006)

    Google Scholar 

  27. Lütkenhaus, N.: Security against individual attacks for realistic quantum key distribution. Physical Review A 61(5), 052304 (2000)

    Article  Google Scholar 

  28. Makarov, V., Hjelme, D.R.: Faked states attack on quantum cryptosystems. Journal of Modern Optics 52, 691–705 (2005)

    Article  Google Scholar 

  29. Makarov, V., Anisimov, A., Skaar, J.: Effects of detector efficiency mismatch on security of quantum cryptosystems. Physical Review A 74, 022313 (2006)

    Article  Google Scholar 

  30. Marøy, Ø., Lydersen, L., Skaar, J.: Security of quantum key distribution with arbitrary individual imperfections. Physical Review A 82, 032337 (2010)

    Article  Google Scholar 

  31. Mayers, D.: Unconditional security in quantum cryptography. J. ACM 48(3), 351–406 (2001)

    MathSciNet  Google Scholar 

  32. Mayers, D., Yao, A.: Quantum cryptography with imperfect apparatus. In: FOCS 1998, p. 503 (1998)

    Google Scholar 

  33. Muller, A., Herzog, T., Huttner, B., Tittel, W., Zbinden, H., Gisin, N.: “Plug and play” systems for quantum cryptography. Applied Physics Letters 70(7), 793–795 (1997)

    Article  Google Scholar 

  34. Nambu, Y., Hatanaka, T., Nakamura, K.: Planar lightwave circuits for quantum cryptographic systems. Arxiv:quant-ph/0307074 (2003)

    Google Scholar 

  35. Nambu, Y., Hatanaka, T., Nakamura, K.: BB84 quantum key distribution system based on silica-based planar lightwave circuits. Japanese Journal of Applied Physics 43(8B), L1109–L1110 (2004)

    Article  Google Scholar 

  36. Nambu, Y., Yoshino, K., Tomita, A.: Quantum encoder and decoder for practical quantum key distribution using a planar lightwave circuit. Journal of Modern Optics 55(12), 1953–1970 (2008)

    Article  Google Scholar 

  37. Nazarathy, M., Tselniker, I., Regev, Y., Orenstein, M., Katz, M.: Integrated-optical realizations of quantum key distribution over maximally unbiased bases. IEEE Journal of Selected Topics in Quantum Electronics 12(4), 897–913 (2006)

    Article  Google Scholar 

  38. Nazarathy, M.: Quantum key distribution over a fiber-optic channel by means of pulse position modulation. Opt. Lett. 30(12), 1533–1535 (2005)

    Article  Google Scholar 

  39. Renner, R.: Security of Quantum Key Distribution. Ph.D. thesis, Swiss Federal Institute of Technology, Zurich (2005)

    Google Scholar 

  40. Shor, P.W., Preskill, J.: Simple proof of security of the BB84 quantum key distribution protocol. Physical Review Letters 85(2), 441–444 (2000)

    Article  Google Scholar 

  41. Stucki, D., Gisin, N., Guinnard, O., Ribordy, G., Zbinden, H.: Quantum key distribution over 67 km with a plug&play system. New Journal of Physics 4, 41 (2002)

    Article  Google Scholar 

  42. Takesue, H., Diamanti, E., Honjo, T., Langrock, C., Fejer, M.M., Inoue, K., Yamamoto, Y.: Differential phase shift quantum key distribution experiment over 105km fibre. New Journal of Physics 7, 232 (2005)

    Article  Google Scholar 

  43. Takesue, H., Honjo, T., Kamada, H.: Differential phase shift quantum key distribution using 1.3-μm up-conversion detectors. Japanese Journal of Applied Physics 45, 5757 (2006)

    Article  Google Scholar 

  44. Townsend, P.D.: Secure key distribution system based on quantum cryptography. Electronics Letters 30, 809–811 (1994)

    Article  MathSciNet  Google Scholar 

  45. Waks, E., Takesue, H., Yamamoto, Y.: Security of differential-phase-shift quantum key distribution against individual attacks. Physical Review A (Atomic, Molecular, and Optical Physics) 73(1), 012344 (2006)

    Article  Google Scholar 

  46. Walton, Z.D., Abouraddy, A.F., Sergienko, A.V., Saleh, B.E.A., Teich, M.C.: Decoherence-free subspaces in quantum key distribution. Physical Review Letters 91(8), 087901 (2003)

    Article  Google Scholar 

  47. Yoshino, K., Fujiwara, M., Tanaka, A., Takahashi, S., Nambu, Y., Tomita, A., Miki, S., Yamashita, T., Wang, Z., Sasaki, M., Tajima, A.: High-speed wavelength-division multiplexing quantum key distribution system. Opt. Lett. 37(2), 223–225 (2012)

    Article  Google Scholar 

  48. Zou, X., Qiu, D., Li, L., Wu, L., Li, L.: Semiquantum-key distribution using less than four quantum states. Physical Review A 79(5), 052312 (2009)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Computer Science Department, University of California, Los Angeles, USA

    Ran Gelles

  2. Computer Science Department, Technion, Haifa, Israel

    Tal Mor

Authors
  1. Ran Gelles
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tal Mor
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Research Group on Mathematical Linguistics, Universitat Rovira i Virgili, Avinguda Catalunya, 35, 43002, Tarragona, Spain

    Adrian-Horia Dediu  & Carlos Martín-Vide  & 

  2. Fakultät für Informatik, Otto-von-Guericke-Universität Magdeburg, Universitätsplatz 2, 39106, Magdeburg, Germany

    Bianca Truthe

Rights and permissions

Reprints and permissions

Copyright information

© 2012 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Gelles, R., Mor, T. (2012). On the Security of Interferometric Quantum Key Distribution. In: Dediu, AH., Martín-Vide, C., Truthe, B. (eds) Theory and Practice of Natural Computing. TPNC 2012. Lecture Notes in Computer Science, vol 7505. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-33860-1_12

Download citation

  • DOI: https://doi.org/10.1007/978-3-642-33860-1_12

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-642-33859-5

  • Online ISBN: 978-3-642-33860-1

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics

Search

Navigation