iBet uBet web content aggregator. Adding the entire web to your favor.
iBet uBet web content aggregator. Adding the entire web to your favor.



Link to original content: https://doi.org/10.1007/s10915-020-01191-3
A Mixed Discontinuous Galerkin Method for Linear Elasticity with Strongly Imposed Symmetry | Journal of Scientific Computing Skip to main content
Springer Nature Link
Log in

A Mixed Discontinuous Galerkin Method for Linear Elasticity with Strongly Imposed Symmetry

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

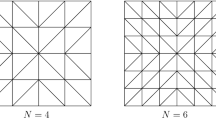
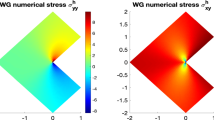
In this paper, we study a mixed discontinuous Galerkin (MDG) method to solve linear elasticity problem with arbitrary order discontinuous finite element spaces in d-dimension (\(d=2,3\)). This method uses polynomials of degree \(k+1\) for the stress and of degree k for the displacement (\(k\ge 0\)). The mixed DG scheme is proved to be well-posed under proper norms. Specifically, we prove that, for any \(k \ge 0\), the \(H(\mathrm{div})\)-like error estimate for the stress and \(L^2\) error estimate for the displacement are optimal. We further establish the optimal \(L^2\) error estimate for the stress provided that the \({\mathcal {P}}_{k+2}-{\mathcal {P}}_{k+1}^{-1}\) Stokes pair is stable and \(k \ge d\). We also provide numerical results of MDG showing that the orders of convergence are actually sharp.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adams, S., Cockburn, B.: A mixed finite element method for elasticity in three dimensions. J. Sci. Comput. 25(3), 515–521 (2005)

    MathSciNet  MATH  Google Scholar 

  2. Amara, M., Thomas, J.-M.: Equilibrium finite elements for the linear elastic problem. Numer. Math. 33(4), 367–383 (1979)

    MathSciNet  MATH  Google Scholar 

  3. Arnold, D., Awanou, G., Winther, R.: Finite elements for symmetric tensors in three dimensions. Math. Comput. 77(263), 1229–1251 (2008)

    MathSciNet  MATH  Google Scholar 

  4. Arnold, D., Awanou, G., Winther, R.: Nonconforming tetrahedral mixed finite elements for elasticity. Math. Models Methods Appl. Sci. 24(04), 783–796 (2014)

    MathSciNet  MATH  Google Scholar 

  5. Arnold, D., Douglas Jr., J., Gupta, C.: A family of higher order mixed finite element methods for plane elasticity. Numer. Math. 45(1), 1–22 (1984)

    MathSciNet  MATH  Google Scholar 

  6. Arnold, D., Falk, R., Winther, R.: Mixed finite element methods for linear elasticity with weakly imposed symmetry. Math. Comput. 76(260), 1699–1723 (2007)

    MathSciNet  MATH  Google Scholar 

  7. Arnold, D., Winther, R.: Mixed finite elements for elasticity. Numer. Math. 92(3), 401–419 (2002)

    MathSciNet  MATH  Google Scholar 

  8. Arnold, D., Winther, R.: Nonconforming mixed elements for elasticity. Math. Models Methods Appl. Sci. 13(03), 295–307 (2003)

    MathSciNet  MATH  Google Scholar 

  9. Arnold, D.N., Brezzi, F., Cockburn, B., Marini, L.D.: Unified analysis of discontinuous Galerkin methods for elliptic problems. SIAM J. Numer. Anal. 39(5), 1749–1779 (2002)

    MathSciNet  MATH  Google Scholar 

  10. Arnold, D.N., Falk, R.S., Winther, R.: Finite element exterior calculus, homological techniques, and applications. Acta Numer. 15, 1–155 (2006)

    MathSciNet  MATH  Google Scholar 

  11. Boffi, D., Brezzi, F., Fortin, M.: Reduced symmetry elements in linear elasticity. Commun. Pure Appl. Anal. 8(1), 95–121 (2009)

    MathSciNet  MATH  Google Scholar 

  12. Boffi, D., Brezzi, F., Fortin, M.: Mixed Finite Element Methods and Applications. Springer Series in Computational Mathematics. Springer, Berlin (2013)

    MATH  Google Scholar 

  13. Brenner, S., Scott, R.: The Mathematical Theory of Finite Element Methods, vol. 15. Springer, Berlin (2007)

    Google Scholar 

  14. Brezzi, F.: On the existence, uniqueness and approximation of saddle-point problems arising from Lagrangian multipliers. Revue française d’automatique, informatique, recherche opérationnelle. Analyse numérique 8(2), 129–151 (1974)

    MathSciNet  MATH  Google Scholar 

  15. Brezzi, F., Douglas Jr., J., Marini, L.D.: Two families of mixed finite elements for second order elliptic problems. Numer. Math. 47(2), 217–235 (1985)

    MathSciNet  MATH  Google Scholar 

  16. Brezzi, F., Fortin, M.: Mixed and Hybrid Finite Element Methods. Springer Series in Computational Mathematics, vol. 15. Springer, Berlin (1991)

    MATH  Google Scholar 

  17. Brezzi, F., Manzini, G., Marini, D., Pietra, P., Russo, A.: Discontinuous Galerkin approximations for elliptic problems. Numer. Methods Partial Differ. Equ. 16(4), 365–378 (2000)

    MathSciNet  MATH  Google Scholar 

  18. Cai, Z., Ye, X.: A mixed nonconforming finite element for linear elasticity. Numer. Methods Partial Differ. Equ. 21(6), 1043–1051 (2005)

    MathSciNet  MATH  Google Scholar 

  19. Castillo, P., Cockburn, B., Perugia, I., Schötzau, D.: An a priori error analysis of the local discontinuous Galerkin method for elliptic problems. SIAM J. Numer. Anal. 38(5), 1676–1706 (2000)

    MathSciNet  MATH  Google Scholar 

  20. Chen, L., Jun, H., Huang, X.: Stabilized mixed finite element methods for linear elasticity on simplicial grids in \({\mathbb{R}}^n\). Comput. Methods Appl. Math. 17(1), 17–31 (2017)

    MathSciNet  MATH  Google Scholar 

  21. Chen, Y., Huang, J., Huang, X., Yifeng, X.: On the local discontinuous Galerkin method for linear elasticity. Math. Probl. Eng. 2010, 759547 (2010). https://doi.org/10.1155/2010/759547

    Article  MathSciNet  MATH  Google Scholar 

  22. Cockburn, B.: Discontinuous Galerkin methods. ZAMM-J. Appl. Math. Mech./Zeitschrift für Angewandte Mathematik und Mechanik: Applied Mathematics and Mechanics 83(11), 731–754 (2003)

    MathSciNet  MATH  Google Scholar 

  23. Cockburn, B., Gopalakrishnan, J., Guzmán, J.: A new elasticity element made for enforcing weak stress symmetry. Math. Comput. 79(271), 1331–1349 (2010)

    MathSciNet  MATH  Google Scholar 

  24. Cockburn, B., Gopalakrishnan, J., Lazarov, R.: Unified hybridization of discontinuous Galerkin, mixed, and continuous Galerkin methods for second order elliptic problems. SIAM J. Numer. Anal. 47(2), 1319–1365 (2009)

    MathSciNet  MATH  Google Scholar 

  25. Cockburn, B., Shu, C.-W.: The local discontinuous Galerkin method for time-dependent convection-diffusion systems. SIAM J. Numer. Anal. 35(6), 2440–2463 (1998)

    MathSciNet  MATH  Google Scholar 

  26. Falk, R.S.: Finite element methods for linear elasticity. In: Brezzi, F., Boffi, D., Demkowicz, L., Duràn, R.G., Falk, R.S., Fortin, M. (eds.) Mixed Finite Elements, Compatibility Conditions, and Applications, pp. 159–194. Springer, Berlin (2008)

    Google Scholar 

  27. Farhloul, M., Fortin, M.: Dual hybrid methods for the elasticity and the stokes problems: a unified approach. Numer. Math. 76(4), 419–440 (1997)

    MathSciNet  MATH  Google Scholar 

  28. Gong, S., Shuonan, W., Jinchao, X.: New hybridized mixed methods for linear elasticity and optimal multilevel solvers. Numer. Math. 141(2), 569–604 (2019)

    MathSciNet  MATH  Google Scholar 

  29. Gopalakrishnan, J., Guzmán, J.: Symmetric nonconforming mixed finite elements for linear elasticity. SIAM J. Numer. Anal. 49(4), 1504–1520 (2011)

    MathSciNet  MATH  Google Scholar 

  30. Gopalakrishnan, J., Guzmán, J.: A second elasticity element using the matrix bubble. IMA J. Numer. Anal. 32(1), 352–372 (2012)

    MathSciNet  MATH  Google Scholar 

  31. Guzman, J., Scott, R.: The Scott–Vogelius finite elements revisited. Math. Comput. 88, 519–529 (2019)

    MathSciNet  MATH  Google Scholar 

  32. Hong, Q., Wang, F., Shuonan, W., Jinchao, X.: A unified study of continuous and discontinuous Galerkin methods. Sci. China Math. 62(1), 1–32 (2019)

    MathSciNet  MATH  Google Scholar 

  33. Hu, J., Zhang, S.: A family of conforming mixed finite elements for linear elasticity on triangular grids (2014). arXiv preprint arXiv:1406.7457

  34. Jun, H.: Finite element approximations of symmetric tensors on simplicial grids in \({\mathbb{R}}^n\): The higher order case. J. Comput. Math. 33(3), 1–14 (2015)

    MathSciNet  Google Scholar 

  35. Jun, H., Zhang, S.Y.: A family of symmetric mixed finite elements for linear elasticity on tetrahedral grids. Sci. China Math. 58(2), 297–307 (2015)

    MathSciNet  MATH  Google Scholar 

  36. Jun, H., Zhang, S.: Finite element approximations of symmetric tensors on simplicial grids in \({\mathbb{R}}^n\): the lower order case. Math. Models Methods Appl. Sci. 26(09), 1649–1669 (2016)

    MathSciNet  MATH  Google Scholar 

  37. Johnson, C., Mercier, B.: Some equilibrium finite element methods for two-dimensional elasticity problems. Numer. Math. 30(1), 103–116 (1978)

    MathSciNet  MATH  Google Scholar 

  38. Qian, Y., Wu, S., Wang, F.: A mixed discontinuous galerkin method with symmetric stress for Brinkman problem based on the velocity-pseudostress formulation (2019). arXiv preprint arXiv:1907.01246

  39. Qiu, W., Demkowicz, L.: Mixed hp-finite element method for linear elasticity with weakly imposed symmetry. Comput. Methods Appl. Mech. Eng. 198(47), 3682–3701 (2009)

    MathSciNet  MATH  Google Scholar 

  40. Qiu, W., Shen, J., Shi, K.: An HDG method for linear elasticity with strong symmetric stresses. Math. Comput. 87(309), 69–93 (2018)

    MathSciNet  MATH  Google Scholar 

  41. Scott, L.R., Vogelius, M.: Norm estimates for a maximal right inverse of the divergence operator in spaces of piecewise polynomials. ESAIM: Math. Model. Numer. Anal. 19(1), 111–143 (1985)

    MathSciNet  MATH  Google Scholar 

  42. Scott, L.R., Zhang, S.: Finite element interpolation of nonsmooth functions satisfying boundary conditions. Math. Comput. 54(190), 483–493 (1990)

    MathSciNet  MATH  Google Scholar 

  43. Soon, S.-C., Cockburn, B., Stolarski, H.K.: A hybridizable discontinuous Galerkin method for linear elasticity. Int. J. Numer. Methods Eng. 80(8), 1058–1092 (2009)

    MathSciNet  MATH  Google Scholar 

  44. Shuonan, W., Gong, S., Jinchao, X.: Interior penalty mixed finite element methods of any order in any dimension for linear elasticity with strongly symmetric stress tensor. Math. Models Methods Appl. Sci. 27(14), 2711–2743 (2017)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics and State Key Laboratory of Multiphase Flow in Power Engineering, Xi’an Jiaotong University, Xi’an, 710049, Shaanxi, China

    Fei Wang

  2. School of Mathematical Sciences, Peking University, Beijing, 100871, China

    Shuonan Wu

  3. Department of Mathematics, Pennsylvania State University, University Park, PA, 16802, USA

    Jinchao Xu

Authors
  1. Fei Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shuonan Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jinchao Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Shuonan Wu.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The work of Fei Wang is partially supported by the National Natural Science Foundation of China (Grant No. 11771350). The work of Shuonan Wu is partially supported by the National Natural Science Foundation of China (Grant No. 11901016) and the startup Grant from Peking University. The work of the Jinchao Xu is partially supported by US Department of Energy Grant DE-SC0014400 and National Science Foundation Grant DMS-1819157.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, F., Wu, S. & Xu, J. A Mixed Discontinuous Galerkin Method for Linear Elasticity with Strongly Imposed Symmetry. J Sci Comput 83, 2 (2020). https://doi.org/10.1007/s10915-020-01191-3

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-020-01191-3

Keywords

Mathematics Subject Classification

Search

Navigation