iBet uBet web content aggregator. Adding the entire web to your favor.
iBet uBet web content aggregator. Adding the entire web to your favor.



Link to original content: https://doi.org/10.1007/s10915-006-9101-0
Arbitrary High-Order Discontinuous Galerkin Schemes for the Magnetohydrodynamic Equations | Journal of Scientific Computing Skip to main content
Springer Nature Link
Log in

Arbitrary High-Order Discontinuous Galerkin Schemes for the Magnetohydrodynamic Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

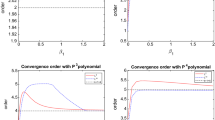
In this paper, we propose a discontinuous Galerkin scheme with arbitrary order of accuracy in space and time for the magnetohydrodynamic equations. It is based on the Arbitrary order using DERivatives (ADER) methodology: the high order time approximation is obtained by a Taylor expansion in time. In this expansion all the time derivatives are replaced by space derivatives via the Cauchy-Kovalevskaya procedure. We propose an efficient algorithm of the Cauchy-Kovalevskaya procedure in the case of the three-dimensional magneto-hydrodynamic (MHD) equations. Parallel to the time derivatives of the conservative variables the time derivatives of the fluxes are calculated. This enables the analytic time integration of the volume integral as well as that of the surface integral of the fluxes through the grid cell interfaces which occur in the discrete equations. At the cell interfaces the fluxes and all their derivatives may jump. Following the finite volume ADER approach the break up of all these jumps into the different waves are taken into account to get proper values of the fluxes at the grid cell interfaces. The approach under considerations is directly based on the expansion of the flux in time in which the leading order term may be any numerical flux calculation for the MHD-equation. Numerical convergence results for these equations up to 7th order of accuracy in space and time are shown.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Dumbser M., Munz C.-D. (2005). Building blocks for arbitrary high order discontinuous Galerkin schemes. J. Sci. Comput. ISSN: 0885-7474 (Paper) 1573-7691 (Online), DOI: 10.1007/s10915-005-9025-0, Issue: Online First.

  2. Toro E., Titarev V. (2003). Solution of the generalized Riemann problem for advection-reaction equations. Proc. Roy. Soc. A: Mathematical, Physical and Engineering Sciences. 458 (2018): 271–281

    Article  MathSciNet  Google Scholar 

  3. Titarev V.A., Toro E.F. (2005). ADER schemes for three-dimensional nonlinear hyperbolic systems. J. Comput. Phys. 204: 715–736

    Article  MATH  MathSciNet  Google Scholar 

  4. Becker J. (2000). Entwicklung eines effizineten Verfahrens zur Lösung hyperbolischer Differentialgleichungen. http://www.freidok.uni-freiburg.de/volltexte/123 (October 2000)

  5. Dumbser M. (2005). Arbitrary High Order Schemes for the Solution of Hyperbolic Conservation Laws in Complex Domains. Shaker Verlag, Aachen

    Google Scholar 

  6. Cockburn B., Shu C.W. (1989). TVB Runge–Kutta local projection discontinuous Galerkin finite element method for conservation laws II: general framework. Math. Comput. 52: 411–435

    Article  MATH  MathSciNet  Google Scholar 

  7. Cockburn B., Shu C.W. (1998). The Runge–Kutta discontinuous Galerkin method for conservation laws V: multidimensional systems. J. Computat. Phys. 141: 199–224

    Article  MATH  MathSciNet  Google Scholar 

  8. Stroud A.H. (1971). Approximate Calculation of Multiple Integrals. Prentice-Hall Inc., Englewood Cliffs, NJ

    MATH  Google Scholar 

  9. Toro E.F., Millington R.C., and Nejad L. A. M. (2001). Towards very high order Godunov schemes, in: Godunov Methods. Theory and Applications, Kluwer/Plenum Academic Publishers, pp. 905–938.

  10. Titarev V.A., Toro E.F. (2002). ADER: arbitrary high order Godunov approach. J. Sci. Comput. 17(1–4): 609–618

    Article  MATH  MathSciNet  Google Scholar 

  11. Dyson R. W. (2001). Technique for very high order nonlinear simulation and validation. Tech. Rep. TM-2001-210985, NASA.

  12. Balsara D.S. (1998). Linearized formulation of the Riemann problem for adiabatic and isothermal magnetohydrodynamics. Astrophys. J. Suppl. Ser. 116: 119–131

    Article  Google Scholar 

  13. Cargo P., Gallice G. (1997). Roe matrices for ideal MHD and systematic construction of Roe matrices for systems of conservation laws. J. Comput. Phys. 136(2): 446–466

    Article  MATH  MathSciNet  Google Scholar 

  14. Roe P.L., Balsara D.S. (1996). Notes on the eigensystem of magnetohydrodynamics. IMA J. Appl. Math. 56(1): 57–67

    MATH  MathSciNet  Google Scholar 

  15. Balsara D.S. (2001). Total variation diminishing scheme for relativistic magnetohydrodynamics. Ap. J. Supp. 132: 1

    Article  Google Scholar 

  16. Ryu D., Jones T.W. (1995). Numerical magnetohydrodynamics in astrophysics: algorithm and tests for one-dimensional flow. Astrophys J. 442: 228–258

    Article  Google Scholar 

  17. Dai W., Woodward P. R. (1994). Extension of the piecewise parabolic method to multidimensional ideal magnetohydrodynamics. J. Comput. Phys. 115(2): 485–514

    Article  MATH  MathSciNet  Google Scholar 

  18. Balsara D. S., A sub-cell based indicator for troubled zones in RKDG schemes and a novel class of hybrid RKDG+HWENO schemes. ArXiv Physics e-prints arXiv:physics/0411160, Provided by the Smithsonian/NASA Astrophysics Data

  19. Qiu J., Shu C.W. (2003). Hermite WENO schemes and their application as limiters for Runge–Kutta discontinuous Galerkin method: one-dimensional case. J. Comput. Phys. 193: 115–135

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut für Aerodynamik und Gasdynamik, University of Stuttgart, Pfaffenwaldring 21, 70569, Stuttgart, Germany

    Arne Taube, Michael Dumbser & Claus-Dieter Munz

  2. Department of Physics, University of Notre Dame, Notre Dame, IN, 46556, USA

    Dinshaw S. Balsara

Authors
  1. Arne Taube
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Michael Dumbser
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dinshaw S. Balsara
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Claus-Dieter Munz
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Arne Taube.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Taube, A., Dumbser, M., Balsara, D.S. et al. Arbitrary High-Order Discontinuous Galerkin Schemes for the Magnetohydrodynamic Equations. J Sci Comput 30, 441–464 (2007). https://doi.org/10.1007/s10915-006-9101-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-006-9101-0

Keywords

Search

Navigation