iBet uBet web content aggregator. Adding the entire web to your favor.
iBet uBet web content aggregator. Adding the entire web to your favor.



Link to original content: https://doi.org/10.1007/s10485-009-9216-1
Thoughts on Quotient-fine Nearness Frames | Applied Categorical Structures Skip to main content
Springer Nature Link
Log in

Thoughts on Quotient-fine Nearness Frames

  • Published:
Applied Categorical Structures Aims and scope Submit manuscript

Abstract

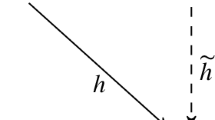
We characterize nearness frames whose completions are fine (we call them quotient-fine), and show that the subcategory QfNFrm they form is reflective in the category of strong nearness frames. The resulting functor commutes with the completion functor. QfNFrm is isomorphic to the subcategory of the functor category (RegFrm)2 given by the dense onto \(h\colon M\to L\), where 2 denotes the category with only two objects and exactly one morphism between them.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Banaschewski, B.: Uniform completion in pointfree topology. In: Topological and Algebraic Structures in Fuzzy Sets, vol. 20, pp. 19–56. Trends Log. Stud. Log. Libr. Kluwer, Dordrecht (2003)

    Google Scholar 

  2. Banaschewski, B., Hong, S.S.: Filters and strict extensions of frames. Kyungpook Math. J. 39, 215–230 (1999)

    MathSciNet  MATH  Google Scholar 

  3. Banaschewski, B., Hong, S.S.: General filters and strict extensions in pointfree topology. Kyungpook Math. J. 42, 273–283 (2002)

    MathSciNet  MATH  Google Scholar 

  4. Banaschewski, B., Hong, S.S., Pultr, A.: On the completion of nearness frames. Quaest. Math. 28, 19–37 (1998)

    Article  MathSciNet  Google Scholar 

  5. Banaschewski, B., Pultr, A.: Paracompactness revisited. Appl. Categ. Struct. 1, 181–190 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  6. Banaschewski, B., Pultr, A.: Cauchy points of uniform and nearness frames. Quaest. Math. 19, 107–127 (1996)

    Article  MathSciNet  Google Scholar 

  7. Bentley, H.L., Herrlich, H.: Extensions of topological spaces. In: Franklin, S.P., Smith-Thomas, B.V. (eds.) Topology, Proceedings of the Memphis State University Conference, 1975, pp. 129–184. Marcel Dekker, New York (1976)

    Google Scholar 

  8. Herrlich, H., Strecker, G.E.: Category Theory. Allyn and Bacon, Boston (1973)

    MATH  Google Scholar 

  9. Johnstone, P.T.: Stone Spaces. Cambridge Univ. Press, Cambridge (1982)

    MATH  Google Scholar 

  10. Picado, J., Pultr, A.: Locales treated mostly in a covariant way. In: Textos de Matemática. Série B [Texts in Mathematics. Series B], vol. 41, xii+104 pp. Universidade de Coimbra, Departamento de Matemática, Coimbra (2008)

    Google Scholar 

  11. Zenk, E.R.: Monocoreflections of completely regular frames. Appl. Categ. Struct. 15, 209–222 (2007)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, University of South Africa, P.O. Box 392, 0003, Unisa, South Africa

    Themba Dube

  2. Department of Mathematics, University of Namibia, P.O. Box 13301, Windhoek, Namibia

    Martin M. Mugochi

Authors
  1. Themba Dube
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Martin M. Mugochi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Themba Dube.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Dube, T., Mugochi, M.M. Thoughts on Quotient-fine Nearness Frames. Appl Categor Struct 19, 511–521 (2011). https://doi.org/10.1007/s10485-009-9216-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10485-009-9216-1

Keywords

Mathematics Subject Classifications (2000)

Search

Navigation